Saint Petersburg Paradox

Consider a game in which a player bets on whether a given Toss of a Coin will turn up heads or tails. If he bets $1 that heads will turn up on the first throw, $2 that heads will turn up on the second throw (if it did not turn up on the first), $4 that heads will turn up on the third throw, etc., his expected payoff is

$${\textstyle{1\over 2}}(1)+{\textstyle{1\over 4}}(2)+{\textst... ...}+{\textstyle{1\over 2}}+{\textstyle{1\over 2}}+\ldots=\infty.$$

Apparently, the player can be in the hole by any amount of money and still come out ahead in the end. This Paradox was first proposed by Daniel Bernoulli.

The paradox arises as a result of muddling the distinction between the amount of the final payoff and the net amount won in the game. It is misleading to consider the payoff without taking into account the amount lost on previous bets, as can be shown as follows. At the time the player first wins (say, on the $n$th toss), he will have lost

$$\sum_{k=1}^{n-1} 2^{k-1}=2^{n-1}-1$$

dollars. In this toss, however, he wins $2^{n-1}$ dollars. This means that the net gain for the player is a whopping $1, no matter how many tosses it takes to finally win. As expected, the large payoff after a long run of tails is exactly balanced by the large amount that the player has to invest.

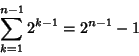
In fact, by noting that the probability of winning on the $n$th toss is $1/2^n$, it can be seen that the probability distribution for the number of tosses needed to win is simply a Geometric Distribution with $p=1/2$.

See also Coin Tossing, Gambler's Ruin, Geometric Distribution, Martingale

References

Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, pp. 44-45, 1987.

Gardner, M. The Scientific American Book of Mathematical Puzzles & Diversions. New York: Simon and Schuster, pp. 51-52, 1959.

Kamke, E. Einführung in die Wahrscheinlichkeitstheorie. Leipzig, Germany, pp. 82-89, 1932.

Keynes, J. M. K. ``The Application of Probability to Conduct.'' In The World of Mathematics, Vol. 2 (Ed. K. Newman). Redmond, WA: Microsoft Press, 1988.

Kraitchik, M. ``The Saint Petersburg Paradox.'' §6.18 in Mathematical Recreations. New York: W. W. Norton, pp. 138-139, 1942.

Todhunter, I. §391 in History of the Mathematical Theory of Probability. New York: Chelsea, p. 221, 1949.