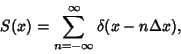The 1-D sampling function is given by
where  is the Dirac Delta Function. The 2-D version is
is the Dirac Delta Function. The 2-D version is
which can be weighted to
where  is a reliability weight,
is a reliability weight, 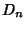 is a density weight (Weighting Function), and
is a density weight (Weighting Function), and  is a taper.
is a taper.
See also Shah Function, Sinc Function
© 1996-9 Eric W. Weisstein
1999-05-26