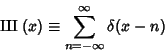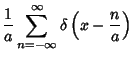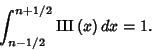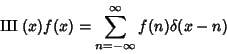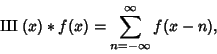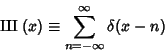 |
(1) |
where 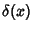 is the Delta Function, so
is the Delta Function, so
 for
for
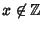 (i.e.,
(i.e., 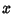 not an Integer). The
shah function obeys the identities
not an Integer). The
shah function obeys the identities
for 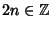 (i.e.,
(i.e., 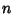 a half-integer).
a half-integer).
It is normalized so that
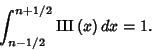 |
(5) |
The ``sampling property'' is
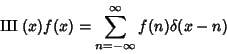 |
(6) |
and the ``replicating property'' is
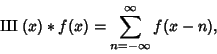 |
(7) |
where  denotes Convolution.
denotes Convolution.
See also Convolution, Delta Function, Impulse Pair
© 1996-9 Eric W. Weisstein
1999-05-26