|
|
|
Let a general Theta Function be defined as
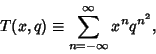
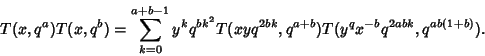
See also Blecksmith-Brillhart-Gerst Theorem, Jacobi Triple Product, Ramanujan Theta Functions
References
Borwein, J. M. and Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity.
New York: Wiley, p. 111, 1987.
Tannery, J. and Molk, J. Elements de la Théorie des Fonctions Elliptiques, 4 vols. Paris: Gauthier-Villars et fils, 1893-1902.