|
|
|
If a random variable ![]() has a Chi-Squared Distribution with
has a Chi-Squared Distribution with ![]() degrees of
freedom (
degrees of
freedom (![]() ) and a random variable
) and a random variable ![]() has a Chi-Squared Distribution
with
has a Chi-Squared Distribution
with ![]() degrees of freedom (
degrees of freedom (![]() ), and
), and ![]() and
and ![]() are independent, then
are independent, then
| (1) |
 |
(2) |
| (3) | |||
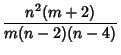 |
(4) | ||
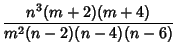 |
(5) | ||
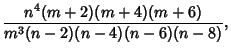 |
(6) |
 |
(7) | ||
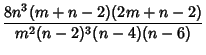 |
(8) | ||
 |
(9) |
| (10) |
| (11) | |||
 |
(12) | ||
 |
(13) | ||
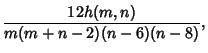 |
(14) |
| (15) |
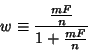 |
(16) |
See also Beta Distribution, Chi-Squared Distribution, Student's t-Distribution
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 536, 1987.
|
|
|
© 1996-9 Eric W. Weisstein