|
|
|
Whittaker and Watson (1990, p. 403) define the internal and external spheroidal wavefunctions as
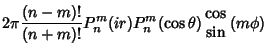 |
|||
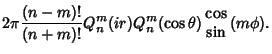 |
See also Ellipsoidal Harmonic, Oblate Spheroidal Wave Function, Prolate Spheroidal Wave Function, Spherical Harmonic
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Spheroidal Wave Functions.'' Ch. 21 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, pp. 751-759, 1972.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 642-644, 1953.
Whittaker, E. T. and Watson, G. N. A Course in Modern Analysis, 4th ed. Cambridge, England:
Cambridge University Press, 1990.