Standard Deviation

The standard deviation is defined as the Square Root of the Variance,

$$\sigma = \sqrt{\left\langle{x^2}\right\rangle{}-\left\langle{x}\right\rangle{}^2}=\sqrt{\mu_2'-\mu^2},$$ (1)

where $\mu=\left\langle{x}\right\rangle{}$ is the Mean and $\mu_2'=\left\langle{x^2}\right\rangle{}$ is the second Moment about 0. The variance $\sigma^2$ is equal to the second Moment about the Mean,

$$\sigma^2=\mu_2.$$ (2)

The square root of the Sample Variance is the ``sample'' standard deviation,

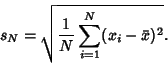$$s_N=\sqrt{{1\over N}\sum_{i=1}^N (x_i-\bar x)^2}.$$ (3)

It is a Biased Estimator of the population standard deviation. As unbiased Estimator is given by

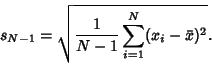$$s_{N-1}=\sqrt{{1\over N-1}\sum_{i=1}^N (x_i-\bar x)^2}.$$ (4)

Physical scientists often use the term Root-Mean-Square as a synonym for standard deviation when they refer to the Square Root of the mean squared deviation of a signal from a given baseline or fit.

See also Mean, Moment, Root-Mean-Square, Sample Variance, Standard Error, Variance