|
|
|
Let ![]() be a set of
be a set of ![]() elements together with a set
elements together with a set ![]() of 3-subset (triples) of
of 3-subset (triples) of ![]() such that every 2-Subset of
such that every 2-Subset of
![]() occurs in exactly one triple of
occurs in exactly one triple of ![]() . Then
. Then ![]() is called a Steiner triple system and is a special case of a Steiner
System with
is called a Steiner triple system and is a special case of a Steiner
System with ![]() and
and ![]() . A Steiner triple system
. A Steiner triple system
![]() of order
of order ![]() exists Iff
exists Iff
![]() (Kirkman 1847). In addition, if Steiner triple systems
(Kirkman 1847). In addition, if Steiner triple systems ![]() and
and ![]() of orders
of orders ![]() and
and ![]() exist, then so does a
Steiner triple system
exist, then so does a
Steiner triple system ![]() of order
of order ![]() (Ryser 1963, p. 101).
(Ryser 1963, p. 101).
Examples of Steiner triple systems ![]() of small orders
of small orders ![]() are
are
The number of nonisomorphic Steiner triple systems ![]() of orders
of orders ![]() , 9, 13, 15, 19, ... (i.e.,
, 9, 13, 15, 19, ... (i.e., ![]() ) are 1,
1, 2, 80,
) are 1,
1, 2, 80,
![]() , ... (Colbourn and Dinitz 1996, pp. 14-15; Sloane's A030129).
, ... (Colbourn and Dinitz 1996, pp. 14-15; Sloane's A030129). ![]() is the same as the
finite Projective Plane of order 2.
is the same as the
finite Projective Plane of order 2. ![]() is a finite Affine Plane which can be constructed from the array
is a finite Affine Plane which can be constructed from the array
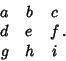
See also Hadamard Matrix, Kirkman Triple System, Steiner Quadruple System, Steiner System
References
Colbourn, C. J. and Dinitz, J. H. (Eds.) ``Steiner Triple Systems.''
§4.5 in CRC Handbook of Combinatorial Designs. Boca Raton, FL: CRC Press, pp.14-15 and 70, 1996.
Kirkman, T. P. ``On a Problem in Combinatorics.'' Cambridge Dublin Math. J. 2, 191-204, 1847.
Lindner, C. C. and Rodger, C. A. Design Theory. Boca Raton, FL: CRC Press, 1997.
Ryser, H. J. Combinatorial Mathematics. Buffalo, NY: Math. Assoc. Amer., pp. 99-102, 1963.
Sloane, N. J. A. Sequence
A030129
in ``The On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html.
Stinson, D. R. and Ferch, H. ``2000000 Steiner Triple Systems of Order 19.'' Math. Comput. 44, 533-535, 1985.
Tonchev, V. D. and Weishaar, R. S. ``Steiner Triple Systems of Order 15 and Their Codes.'' J. Stat. Plan. Inference 58,
207-216, 1997.
|
|
|
© 1996-9 Eric W. Weisstein