Steiner Quadruple System

A Steiner quadruple system is a Steiner System $S(t=3,k=4,v)$, where $S$ is a $v$-set and $B$ is a collection of $k$-sets of $S$ such that every $t$-subset of $S$ is contained in exactly one member of $B$. Barrau (1908) established the uniqueness of $S(3,4,8)$,

$$\matrix{ 1 & 2 & 4 & 8\cr 2 & 3 & 5 & 8\cr 3 & 4 & 6 & 8\cr ... ...& 3 & 6\cr 2 & 3 & 4 & 7\cr 1 & 3 & 4 & 5\cr 2 & 4 & 5 & 6\cr}$$

and $S(3,4,10)$

$$\matrix{ 1 & 2 & 4 & 5\cr 2 & 3 & 5 & 6\cr 3 & 4 & 6 & 7\cr ... ... 7 & 9\cr 2 & 5 & 8 & 0\cr 1 & 3 & 6 & 9\cr 2 & 4 & 7 & 0\cr}.$$

Fitting (1915) subsequently constructed the cyclic systems $S(3,4,26)$ and $S(3,4,34)$, and Bays and de Weck (1935) showed the existence of at least one $S(3,4,14)$. Hanani (1960) proved that a Necessary and Sufficient condition for the existence of an $S(3,4,v)$ is that $v\equiv 2$ or 4 (mod 6).

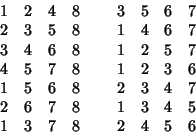
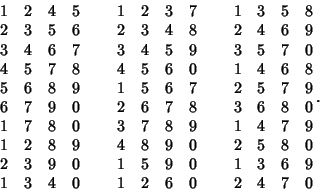
The number of nonisomorphic steiner quadruple systems of orders 8, 10, 14, and 16 are 1, 1, 4 (Mendelsohn and Hung 1972), and at least 31,021 (Lindner and Rosa 1976).

See also Steiner System, Steiner Triple System

References

Barrau, J. A. ``On the Combinatory Problem of Steiner.'' K. Akad. Wet. Amsterdam Proc. Sect. Sci. 11, 352-360, 1908.

Bays, S. and de Weck, E. ``Sur les systèmes de quadruples.'' Comment. Math. Helv. 7, 222-241, 1935.

Fitting, F. ``Zyklische Lösungen des Steiner'schen Problems.'' Nieuw. Arch. Wisk. 11, 140-148, 1915.

Hanani, M. ``On Quadruple Systems.'' Canad. J. Math. 12, 145-157, 1960.

Lindner, C. L. and Rosa, A. ``There are at Least 31,021 Nonisomorphic Steiner Quadruple Systems of Order 16.'' Utilitas Math. 10, 61-64, 1976.

Lindner, C. L. and Rosa, A. ``Steiner Quadruple Systems--A Survey.'' Disc. Math. 22, 147-181, 1978.

Mendelsohn, N. S. and Hung, S. H. Y. ``On the Steiner Systems $S(3,4,14)$ and $S(4,5,15)$.'' Utilitas Math. 1, 5-95, 1972.