|
|
|
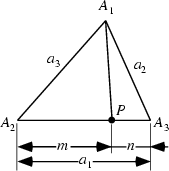
Let a Cevian ![]() be drawn on a Triangle
be drawn on a Triangle
![]() , and denote the lengths
, and denote the lengths
![]() and
and
![]() , with
, with ![]() . Then
. Then
References
Altshiller-Court, N. ``Stewart's Theorem.'' §6B in
College Geometry: A Second Course in Plane Geometry for Colleges and Normal Schools, 2nd ed., rev. enl.
New York: Barnes and Noble, pp. 152-153, 1952.
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., p. 6, 1967.