Integrals of the form
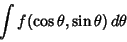 |
(1) |
can be solved by making the substitution
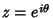 so that
so that
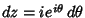 and expressing
and expressing
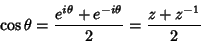 |
(2) |
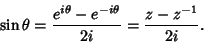 |
(3) |
The integral can then be solved by Contour Integration.
Alternatively, making the substitution
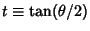 transforms (1) into
transforms (1) into
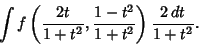 |
(4) |
The following table gives trigonometric substitutions which can be used to transform integrals involving
square roots.
See also Hyperbolic Substitution
© 1996-9 Eric W. Weisstein
1999-05-26
![]() transforms (1) into
transforms (1) into