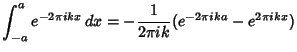An Apodization Function
 |
(1) |
having Instrument Function
The peak (in units of 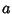 ) is 2. The extrema are given by letting
) is 2. The extrema are given by letting
 and solving
and solving
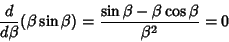 |
(3) |
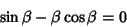 |
(4) |
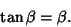 |
(5) |
Solving this numerically gives 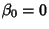 ,
,
 ,
,
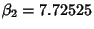 , ...for the first few solutions. The
second of these is the peak Positive sidelobe, and the third is the peak Negative sidelobe. As a fraction of the peak,
they are 0.128375 and
, ...for the first few solutions. The
second of these is the peak Positive sidelobe, and the third is the peak Negative sidelobe. As a fraction of the peak,
they are 0.128375 and 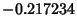 . The Full Width at Half Maximum is found by setting
. The Full Width at Half Maximum is found by setting 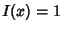
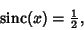 |
(6) |
and solving for 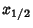 , yielding
, yielding
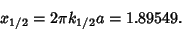 |
(7) |
Therefore, with 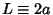 ,
,
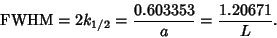 |
(8) |
See also Apodization Function
© 1996-9 Eric W. Weisstein
1999-05-26