|
|
|
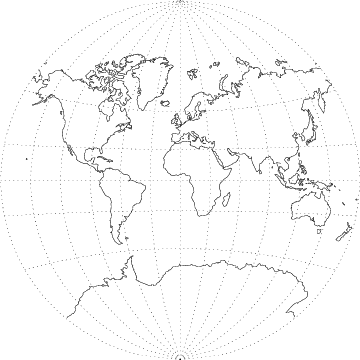
A Map Projection given by the transformation
![$\displaystyle \mathop{\rm sgn}\nolimits (\lambda-\lambda_0){\pi[A(G-P^2)+\sqrt{A^2(G-P^2)^2-(P^2+A^2)(G^2-P^2)}\,]\over P^2+A^2}$](v_89.gif) |
(1) | ||
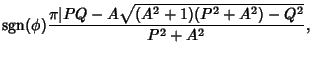 |
(2) |
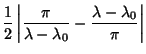 |
(3) | ||
 |
(4) | ||
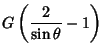 |
(5) | ||
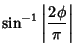 |
(6) | ||
| (7) |
![$\displaystyle \mathop{\rm sgn}\nolimits (y)\pi\left[{-m_1\cos(\theta_1+{\textstyle{1\over 3}}\pi)-{c_2\over 3c_3}}\right]$](v_102.gif) |
(8) | ||
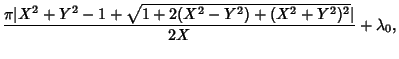 |
|||
| (9) |
where
| (10) | |||
| (11) | |||
| (12) | |||
| (13) | |||
| (14) | |||
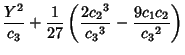 |
(15) | ||
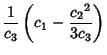 |
(16) | ||
| (17) | |||
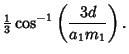 |
(18) |
References
Snyder, J. P. Map Projections--A Working Manual. U. S. Geological Survey Professional Paper 1395.
Washington, DC: U. S. Government Printing Office, pp. 239-242, 1987.
|
|
|
© 1996-9 Eric W. Weisstein