|
|
|
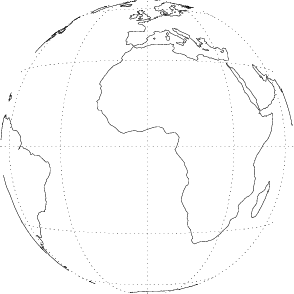
A Map Projection given by the transformation equations
| (1) | |||
| (2) |
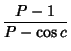 |
(3) | ||
| (4) |
References
Snyder, J. P. Map Projections--A Working Manual. U. S. Geological Survey Professional Paper 1395.
Washington, DC: U. S. Government Printing Office, pp. 173-178, 1987.