Weingarten Equations

The Weingarten equations express the derivatives of the Normal using derivatives of the position vector. Let ${\bf x}:U\to\Bbb{R}^3$ be a Regular Patch, then the Shape Operator of x is given in terms of the basis $\{{\bf x}_u, {\bf x}_v\}$ by

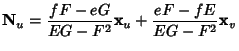$\displaystyle -S({\bf x}_u)$	$\textstyle =$	$\displaystyle {\bf N}_u={fF-eG\over EG-F^2}{\bf x}_u+{eF-fE\over EG-F^2}{\bf x}_v$	(1)
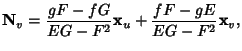$\displaystyle -S({\bf x}_v)$	$\textstyle =$	$\displaystyle {\bf N}_v={gF-fG\over EG-F^2}{\bf x}_u+{fF-gE\over EG-F^2}{\bf x}_v,$	(2)

where ${\bf N}$ is the Normal Vector,

, and

the coefficients of the first Fundamental Form

$\begin{displaymath} ds^2=E\,du^2+2F\,du\,dv+G\,dv^2, \end{displaymath}$

(3)

and

, and

the coefficients of the second Fundamental Form given by

$\displaystyle e$	$\textstyle =$	$\displaystyle -{\bf N}_u\cdot{\bf x}_u={\bf N}\cdot{\bf x}_{uu}$	(4)
$\displaystyle f$	$\textstyle =$	$\displaystyle -{\bf N}_v\cdot{\bf x}_u={\bf N}\cdot{\bf x}_{uv}$
	$\textstyle =$	$\displaystyle {\bf N}_{vu}\cdot{\bf x}_{vu}=-{\bf N}_u\cdot{\bf x}_v$	(5)
$\displaystyle g$	$\textstyle =$	$\displaystyle -{\bf N}_v\cdot{\bf x}_v={\bf N}\cdot{\bf x}_{vv}.$	(6)

See also Fundamental Forms, Shape Operator

References

Gray, A. ``Calculation of the Shape Operator.'' §14.3 in Modern Differential Geometry of Curves and Surfaces. Boca Raton, FL: CRC Press, pp. 274-277, 1993.