|
|
|
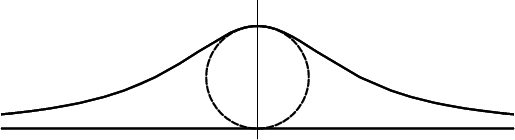
A curve studied and named ``versiera'' (Italian for ``she-devil'' or ``witch'') by Maria Agnesi in 1748 in her book Istituzioni Analitiche (MacTutor Archive). It is also known as Cubique d'Agnesi or
Agnésienne. Some suggest that Agnesi confused an old Italian word meaning ``free to move'' with
another meaning ``witch.'' The curve had been studied earlier by Fermat ![]() and Guido Grandi in 1703.
and Guido Grandi in 1703.
It is the curve obtained by drawing a line from the origin through the Circle of radius ![]() (
(![]() ), then picking the
point with the
), then picking the
point with the ![]() coordinate of the intersection with the circle and the
coordinate of the intersection with the circle and the ![]() coordinate of the intersection of the extension
of line
coordinate of the intersection of the extension
of line ![]() with the line
with the line ![]() . The curve has Inflection Points at
. The curve has Inflection Points at ![]() . The line
. The line ![]() is an Asymptote to the curve.
is an Asymptote to the curve.
In parametric form,
| (1) | |||
| (2) |
| (3) | |||
| (4) |
| (5) |
See also Lamé Curve
References
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 90-93, 1972.
Lee, X. ``Witch of Agnesi.''
http://www.best.com/~xah/SpecialPlaneCurves_dir/WitchOfAgnesi_dir/witchOfAgnesi.html
MacTutor History of Mathematics Archive. ``Witch of Agnesi.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Witch.html.
Yates, R. C. ``Witch of Agnesi.'' A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 237-238, 1952.
|
|
|
© 1996-9 Eric W. Weisstein