|
|
|
A number written as a sum of nonconsecutive Fibonacci Numbers,
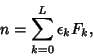
See also Zeckendorf's Theorem
References
Grabner, P. J.; Tichy, R. F.; Nemes, I.; and Pethö, A. ``On the Least Significant Digit of Zeckendorf Expansions.''
Fib. Quart. 34, 147-151, 1996.
Vardi, I. Computational Recreations in Mathematica.
Reading, MA: Addison-Wesley, p. 40, 1991.
Zeckendorf, E. ``Représentation des nombres naturels par une somme des nombres de Fibonacci ou de nombres de
Lucas.'' Bull. Soc. Roy. Sci. Liège 41, 179-182, 1972.