Almost Prime

A number $n$ with prime factorization

$$n=\prod_{i=1}^r {p_i}^{a_i}$$

is called $k$-almost prime when the sum of the Powers $\sum_{i=1}^r a_i=k$. The set of $k$-almost primes is denoted $P_k$.

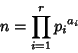
The Primes correspond to the ``1-almost prime'' numbers 2, 3, 5, 7, 11, ... (Sloane's A000040). The 2-almost prime numbers correspond to Semiprimes 4, 6, 9, 10, 14, 15, 21, 22, ... (Sloane's A001358). The first few 3-almost primes are 8, 12, 18, 20, 27, 28, 30, 42, 44, 45, 50, 52, 63, 66, 68, 70, 75, 76, 78, 92, 98, 99, ... (Sloane's A014612). The first few 4-almost primes are 16, 24, 36, 40, 54, 56, 60, 81, 84, 88, 90, 100, ... (Sloane's A014613). The first few 5-almost primes are 32, 48, 72, 80, ... (Sloane's A014614).

See also Chen's Theorem, Prime Number, Semiprime

References

Sloane, N. J. A. Sequences A014612, A014613, A014614, A000040/M0652, and A001358/M3274 in ``An On-Line Version of the Encyclopedia of Integer Sequences.'' http://www.research.att.com/~njas/sequences/eisonline.html.