|
|
|
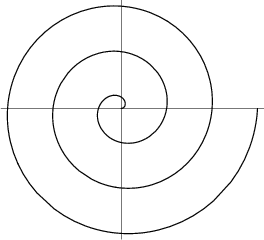
An Archimedean Spiral with Polar equation
Archimedes' spiral can be used for Compass and Straightedge division of an Angle into ![]() parts
(including Angle Trisection) and can also be used for Circle Squaring. In addition, the curve
can be used as a cam to convert uniform circular motion into uniform linear motion. The cam consists of one arch of the
spiral above the x-Axis together with its reflection in the x-Axis. Rotating this with uniform
angular velocity
parts
(including Angle Trisection) and can also be used for Circle Squaring. In addition, the curve
can be used as a cam to convert uniform circular motion into uniform linear motion. The cam consists of one arch of the
spiral above the x-Axis together with its reflection in the x-Axis. Rotating this with uniform
angular velocity ![]() about its center will result in uniform linear motion of the point where
it crosses the y-Axis.
about its center will result in uniform linear motion of the point where
it crosses the y-Axis.
See also Archimedean Spiral
References
Gardner, M. The Unexpected Hanging and Other Mathematical Diversions. Chicago, IL: Chicago University Press,
pp. 106-107, 1991.
Gray, A. Modern Differential Geometry of Curves and Surfaces.
Boca Raton, FL: CRC Press, pp. 69-70, 1993.
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 186-187, 1972.
Lockwood, E. H. A Book of Curves. Cambridge, England: Cambridge University Press, pp. 173-164, 1967.