|
|
|
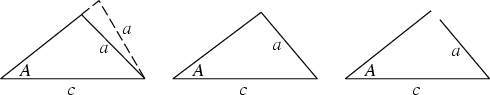
Specifying two adjacent side lengths ![]() and
and ![]() of a Triangle (taking
of a Triangle (taking ![]() ) and one Acute
Angle
) and one Acute
Angle ![]() opposite
opposite ![]() does not, in general, uniquely determine a triangle. If
does not, in general, uniquely determine a triangle. If ![]() , there are two possible
Triangles satisfying the given conditions. If
, there are two possible
Triangles satisfying the given conditions. If ![]() , there is one possible Triangle. If
, there is one possible Triangle. If
![]() , there are no possible Triangles. Remember: don't try to prove congruence with the ASS
theorem or you will make make an ASS out of yourself.
, there are no possible Triangles. Remember: don't try to prove congruence with the ASS
theorem or you will make make an ASS out of yourself.
See also AAA Theorem, AAS Theorem, SAS Theorem, SSS Theorem, Triangle