|
|
|
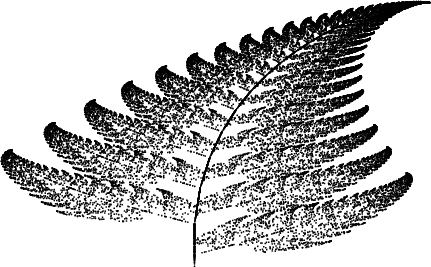
The Attractor of the Iterated Function System given by the set of ``fern functions''
![$\displaystyle \left[\begin{array}{cc}0.85 & 0.04\\ -0.04 & 0.85\end{array}\rig...
...c}x\\ y\end{array}\right]+\left[\begin{array}{c}0.00\\ 1.60\end{array}\right]$](b_151.gif) |
(1) | ||
![$\displaystyle \left[\begin{array}{cc}-0.15 & 0.28\\ 0.26 & 0.24\end{array}\rig...
...c}x\\ y\end{array}\right]+\left[\begin{array}{c}0.00\\ 0.44\end{array}\right]$](b_153.gif) |
(2) | ||
![$\displaystyle \left[\begin{array}{cc}0.20 & -0.26\\ 0.23 & 0.22\end{array}\rig...
...c}x\\ y\end{array}\right]+\left[\begin{array}{c}0.00\\ 1.60\end{array}\right]$](b_155.gif) |
(3) | ||
![$\displaystyle \left[\begin{array}{cc}0.00 & 0.00\\ 0.00 & 0.16\end{array}\right]\left[\begin{array}{c}x\\ y\end{array}\right]$](b_157.gif) |
(4) |
See also Dynamical System, Fractal, Iterated Function System
References
Barnsley, M. Fractals Everywhere, 2nd ed. Boston, MA: Academic Press, pp. 86, 90, 102 and Plate 2, 1993.
Gleick, J. Chaos: Making a New Science. New York: Penguin Books, p. 238, 1988.
Wagon, S. ``Biasing the Chaos Game: Barnsley's Fern.'' §5.3 in Mathematica in Action.
New York: W. H. Freeman, pp. 156-163, 1991.