Binomial Theorem

The theorem that, for Integral Positive $n$,

$$(x+a)^n = \sum_{k=0}^n {n!\over k!(n-k)!} x^k a^{n-k} = \sum_{k=0}^n {n\choose k} x^k a^{n-k},$$

the so-called Binomial Series, where ${n\choose k}$ are Binomial Coefficients. The theorem was known for the case $n=2$ by Euclid around 300 BC, and stated in its modern form by Pascal in 1665. Newton (1676) showed that a similar formula (with Infinite upper limit) holds for Negative Integral $n$,

$$(x+a)^{-n}=\sum_{k=0}^\infty {-n\choose k} x^k a^{-n-k},$$

the so-called Negative Binomial Series, which converges for $\vert x\vert>\vert a\vert$.

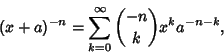
See also Binomial Coefficient, Binomial Series, Cauchy Binomial Theorem, Chu-Vandermonde Identity, Logarithmic Binomial Formula, Negative Binomial Series, q-Binomial Theorem, Random Walk

References

Abramowitz, M. and Stegun, C. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 10, 1972.

Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 307-308, 1985.

Conway, J. H. and Guy, R. K. ``Choice Numbers Are Binomial Coefficients.'' In The Book of Numbers. New York: Springer-Verlag, pp. 72-74, 1996.

Coolidge, J. L. ``The Story of the Binomial Theorem.'' Amer. Math. Monthly 56, 147-157, 1949.

Courant, R. and Robbins, H. ``The Binomial Theorem.'' §1.6 in What is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 16-18, 1996.