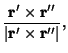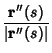Binormal Vector

$\displaystyle {\bf\hat B}$	$\textstyle \equiv$	$\displaystyle {\bf\hat T}\times{\bf\hat N}$	(1)
	$\textstyle =$	$\displaystyle {{\bf r}'\times {\bf r}''\over \vert{\bf r}'\times{\bf r}''\vert},$	(2)

where the unit Tangent Vector 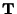${\bf T}$ and unit ``principal'' Normal Vector 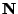${\bf N}$ are defined by

$\displaystyle {\bf\hat T}$	$\textstyle \equiv$	$\displaystyle {{\bf r}'(s)\over \vert{\bf r}'(s)\vert}$	(3)
$\displaystyle {\bf\hat N}$	$\textstyle \equiv$	$\displaystyle {{\bf r}''(s)\over \vert{\bf r}''(s)\vert}$	(4)

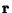Here, ${\bf r}$ is the Radius Vector,

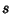is the Arc Length, 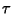$\tau$ is the Torsion, and 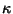$\kappa$ is the Curvature. The binormal vector satisfies the remarkable identity

$\begin{displaymath}[\dot{\bf B},\ddot{\bf B},\raise7.5pt\hbox{.}\mkern0mu\raise7... ...15mu{\bf B}]=\tau^5 {d\over ds}\left({\kappa\over\tau}\right). \end{displaymath}$

(5)

References

Kreyszig, E. ``Binormal. Moving Trihedron of a Curve.'' §13 in Differential Geometry. New York: Dover, p. 36-37, 1991.