A (symmetrical) boundary set of Radius 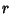 and center
and center 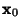 is the set of all points
is the set of all points 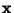 such that
such that
Let 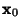 be the Origin. In
be the Origin. In  , the boundary set is then the pair of points
, the boundary set is then the pair of points 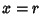 and
and 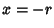 . In
. In
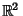 , the boundary set is a Circle. In
, the boundary set is a Circle. In 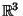 , the boundary set is a Sphere.
, the boundary set is a Sphere.
See also Circle, Disk, Open Set, Sphere
© 1996-9 Eric W. Weisstein
1999-05-26
![]() and center
and center ![]() is the set of all points
is the set of all points ![]() such that
such that