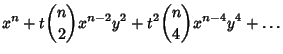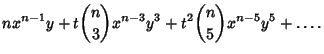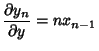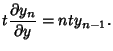One of the Polynomials obtained by taking Powers of the Brahmagupta Matrix. They satisfy the recurrence relation
A list of many others is given by Suryanarayan (1996). Explicitly,
The Brahmagupta Polynomials satisfy
The first few Polynomials are
and
Taking 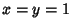 and
and 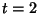 gives
gives 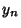 equal to the Pell Numbers and
equal to the Pell Numbers and  equal to half the Pell-Lucas
numbers. The Brahmagupta Polynomials are related to the Morgan-Voyce Polynomials, but the relationship given by Suryanarayan (1996) is incorrect.
equal to half the Pell-Lucas
numbers. The Brahmagupta Polynomials are related to the Morgan-Voyce Polynomials, but the relationship given by Suryanarayan (1996) is incorrect.
References
Suryanarayan, E. R. ``The Brahmagupta Polynomials.'' Fib. Quart. 34, 30-39, 1996.
© 1996-9 Eric W. Weisstein
1999-05-26