|
|
|
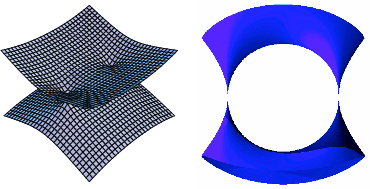
The Quartic Surface obtained by replacing the constant ![]() in the equation of the Cassini Ovals
in the equation of the Cassini Ovals
| (1) |
| (2) |
| (3) |
| (4) |
References
Fischer, G. (Ed.). Mathematical Models from the Collections of Universities and Museums.
Braunschweig, Germany: Vieweg, p. 20, 1986.
Fischer, G. (Ed.). Plate 51 in
Mathematische Modelle/Mathematical Models, Bildband/Photograph Volume.
Braunschweig, Germany: Vieweg, p. 51, 1986.