|
|
|
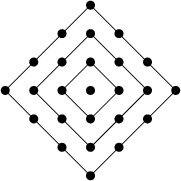
A Centered Polygonal Number consisting of a central dot with four dots around it, and then additional dots in the gaps
between adjacent dots. The general term is ![]() , and the first few such numbers are 1, 5, 13, 25, 41, ...
(Sloane's A001844). Centered square numbers are the sum of two consecutive Square Numbers and are
congruent to 1 (mod 4). The Generating Function giving the centered square numbers is
, and the first few such numbers are 1, 5, 13, 25, 41, ...
(Sloane's A001844). Centered square numbers are the sum of two consecutive Square Numbers and are
congruent to 1 (mod 4). The Generating Function giving the centered square numbers is
See also Centered Pentagonal Number, Centered Polygonal Number, Centered Triangular Number, Square Number
References
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, p. 41, 1996.
Sloane, N. J. A. Sequence
A001844/M3826
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.