A number theoretic function 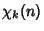 for Positive integral
for Positive integral 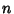 is a character modulo
is a character modulo 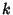 if
if
for all 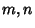 , and
, and
if 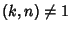 .
. 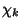 can only assume values which are
can only assume values which are 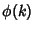 Roots of Unity, where
Roots of Unity, where 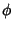 is the Totient Function.
is the Totient Function.
See also Dirichlet L-Series
© 1996-9 Eric W. Weisstein
1999-05-26
![]() for Positive integral
for Positive integral ![]() is a character modulo
is a character modulo ![]() if
if