|
|
|
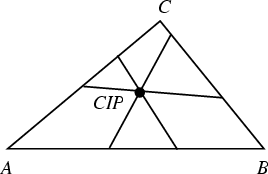
In 1989, P. Yff proved there is a unique configuration of Isoscelizers for a given
Triangle such that all three have the same length. Furthermore, these Isoscelizers
meet in a point called the congruent isoscelizers point, which has Triangle Center Function
See also Isoscelizer
References
Kimberling, C. ``Congruent Isoscelizers Point.''
http://cedar.evansville.edu/~ck6/tcenters/recent/conisos.html.