|
|
|
A variant of the Riemann Integral defined when the Upper and Lower Integrals, taken as limits of the Lower Sum
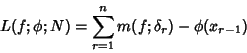
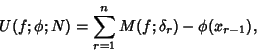
See also Lower Integral, Lower Sum, Riemann Integral, Upper Integral, Upper Sum
References
Kestelman, H. Modern Theories of Integration, 2nd rev. ed. New York: Dover, p. 250, 1960.