|
|
|
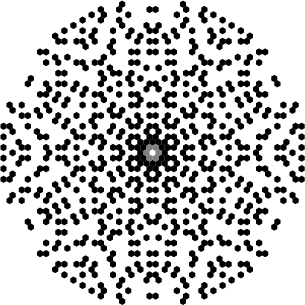
Let ![]() be the Cube Root of unity
be the Cube Root of unity
![]() . Then the Eisenstein primes are
. Then the Eisenstein primes are
References
Cox, D. A. §4A in Primes of the Form
Guy, R. K. ``Gaussian Primes. Eisenstein-Jacobi Primes.'' §A16 in
Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 33-36, 1994.
Wagon, S. ``Eisenstein Primes.'' Mathematica in Action. New York: W. H. Freeman, pp. 278-279, 1991.
![]() : Fermat, Class Field Theory and Complex Multiplication.
New York: Wiley, 1989.
: Fermat, Class Field Theory and Complex Multiplication.
New York: Wiley, 1989.