|
|
|
The Erdös-Selfridge function ![]() is defined as the least integer bigger than
is defined as the least integer bigger than ![]() such that all prime factors
of
such that all prime factors
of
![]() exceed
exceed ![]() (Ecklund et al. 1974). The best lower bound known is
(Ecklund et al. 1974). The best lower bound known is
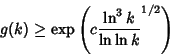
See also Binomial Coefficient, Least Prime Factor
References
Ecklund, E. F. Jr.; Erdös, P.; and Selfridge, J. L. ``A New Function Associated with the prime factors of
Erdös, P.; Lacampagne, C. B.; and Selfridge, J. L. ``Estimates of the Least Prime Factor of a Binomial Coefficient.'' Math. Comput.
61, 215-224, 1993.
Granville, A. and Ramare, O. ``Explicit Bounds on Exponential Sums and the Scarcity of Squarefree Binomial Coefficients.''
Mathematika 43, 73-107, 1996.
Lukes, R. F.; Scheidler, R.; and Williams, H. C. ``Further Tabulation of the Erdös-Selfridge Function.'' Math. Comput. 66,
1709-1717, 1997.
Scheidler, R. and Williams, H. C. ``A Method of Tabulating the Number-Theoretic Function
Sloane, N. J. A. Sequence
A046105
in ``The On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html.
![]() .
Math. Comput. 28, 647-649, 1974.
.
Math. Comput. 28, 647-649, 1974.
![]() .'' Math. Comput. 59, 251-257, 1992.
.'' Math. Comput. 59, 251-257, 1992.