|
|
|
Accelerates the rate of Convergence for an Alternating Series
![\begin{displaymath}
S=\sum_{s=0}^\infty (-1)^s u_s = u_0-u_1+u_2-\ldots-u_{n-1}+\sum_{s=0}^\infty {(-1)^2\over 2^{s+1}} [\Delta^s u_n]
\end{displaymath}](e_2363.gif) |
(1) |
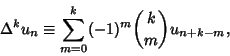 |
(2) |
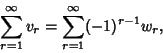 |
(3) |
| (4) |
See also Alternating Series
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 16, 1972.