|
|
|
A technique for Series Convergence Improvement which takes a convergent alternating series
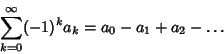 |
(1) |
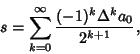 |
(2) |
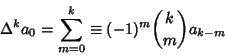 |
(3) |
See also Forward Difference
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 16, 1972.
Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, Feb. 1972.