|
|
|
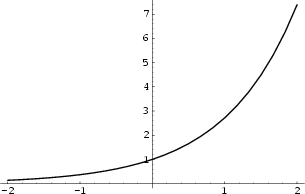
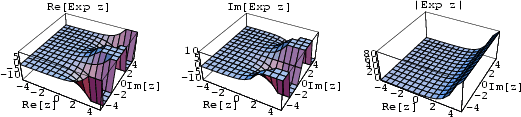
The exponential function is defined by
| (1) |
| (2) |
| (3) |
| (4) |
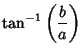 |
(5) | ||
![$\displaystyle \ln\left\{{b\csc \left[{\tan^{-1}\left({b\over a}\right)}\right]}\right\}$](e_2724.gif) |
|||
![$\displaystyle \ln\left\{{a\sec \left[{\tan^{-1}\left({b\over a}\right)}\right]}\right\}.$](e_2725.gif) |
(6) |
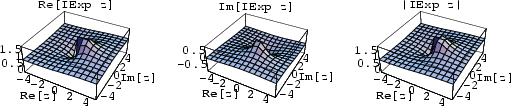
The above plot shows the function ![]() .
.
See also Euler Formula, Exponential Ramp, Fourier Transform--Exponential Function, Sigmoid Function
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Exponential Function.'' §4.2 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 69-71, 1972.
Fischer, G. (Ed.). Plates 127-128 in
Mathematische Modelle/Mathematical Models, Bildband/Photograph Volume.
Braunschweig, Germany: Vieweg, pp. 124-125, 1986.
Spanier, J. and Oldham, K. B. ``The Exponential Function
Yates, R. C. ``Exponential Curves.'' A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 86-97, 1952.
![]() '' and ``Exponentials of Powers
'' and ``Exponentials of Powers
![]() .''
Chs. 26-27 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 233-261, 1987.
.''
Chs. 26-27 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 233-261, 1987.