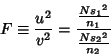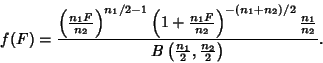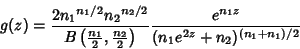 |
(1) |
(Kenney and Keeping 1951). This general distribution includes the Chi-Squared Distribution and Student's t-Distribution as special cases. Let 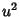 and
and 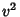 be Independent Unbiased Estimators of the Variance of a Normally Distributed variate. Define
be Independent Unbiased Estimators of the Variance of a Normally Distributed variate. Define
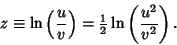 |
(2) |
Then let
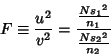 |
(3) |
so that 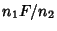 is a ratio of Chi-Squared variates
is a ratio of Chi-Squared variates
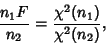 |
(4) |
which makes it a ratio of Gamma Distribution variates,
which is itself a Beta Prime Distribution variate,
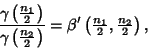 |
(5) |
giving
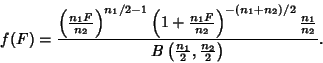 |
(6) |
The Mean is
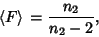 |
(7) |
and the Mode is
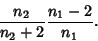 |
(8) |
See also Beta Distribution, Beta Prime Distribution, Chi-Squared Distribution, Gamma Distribution,
Normal Distribution, Student's t-Distribution
References
Kenney, J. F. and Keeping, E. S. Mathematics of Statistics, Pt. 2, 2nd ed. Princeton, NJ: Van Nostrand, pp. 180-181, 1951.
© 1996-9 Eric W. Weisstein
1999-05-26