A four-element vector
![\begin{displaymath}
a^\mu =\left[{\matrix{a^0\cr a^1\cr a^2\cr a^3\cr}}\right],
\end{displaymath}](f_1608.gif) |
(1) |
which transforms under a Lorentz Transformation like the Position Four-Vector. This means it obeys
 |
(2) |
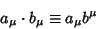 |
(3) |
 |
(4) |
where
 is the Lorentz Tensor. Multiplication of two four-vectors with the Metric
is the Lorentz Tensor. Multiplication of two four-vectors with the Metric
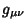 gives products of the form
gives products of the form
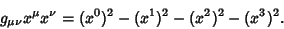 |
(5) |
In the case of the Position Four-Vector,  (where
(where 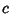 is the speed of light
is the speed of light  )
and this product is an invariant known as the spacetime interval.
)
and this product is an invariant known as the spacetime interval. 
See also Gradient Four-Vector, Lorentz Transformation, Position Four-Vector, Quaternion
References
Morse, P. M. and Feshbach, H. ``The Lorentz Transformation, Four-Vectors, Spinors.'' §1.7 in
Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 93-107, 1953.
© 1996-9 Eric W. Weisstein
1999-05-26
![\begin{displaymath}
a^\mu =\left[{\matrix{a^0\cr a^1\cr a^2\cr a^3\cr}}\right],
\end{displaymath}](f_1608.gif)