The quantity
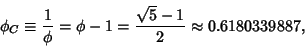 |
(1) |
where 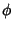 is the Golden Ratio. The golden ratio conjugate is sometimes also called the Silver Ratio.
A quantity similar to the Feigenbaum Constant can be found for the
is the Golden Ratio. The golden ratio conjugate is sometimes also called the Silver Ratio.
A quantity similar to the Feigenbaum Constant can be found for the 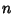 th Continued Fraction representation
th Continued Fraction representation
![\begin{displaymath}[a_0, a_1, a_2, \ldots].
\end{displaymath}](g_1630.gif) |
(2) |
Taking the limit of
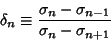 |
(3) |
gives
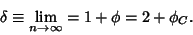 |
(4) |
See also Golden Ratio, Silver Ratio
© 1996-9 Eric W. Weisstein
1999-05-25