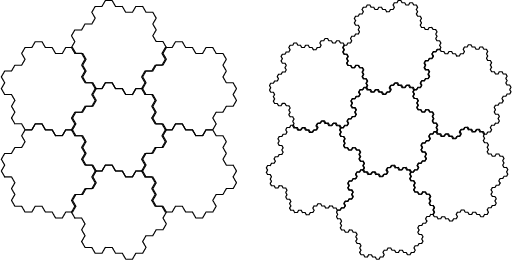
A modification of the Koch Snowflake which has Fractal Dimension
The term ``Gosper island'' was used by Mandelbrot (1977) because this curve bounds the space filled by the
Peano-Gosper Curve; Gosper and Gardner use the term Flowsnake Fractal instead. Gosper islands can
Tile the Plane.
See also Koch Snowflake, Peano-Gosper Curve
References
Mandelbrot, B. B. Fractals: Form, Chance, & Dimension. San Francisco, CA: W. H. Freeman,
Plate 46, 1977.
© 1996-9 Eric W. Weisstein
1999-05-25