|
|
|
The smallest dimension of a Hypercube such that if the lines joining all pairs of corners are two-colored, a
Planar Complete Graph ![]() of one color will be forced. That an answer exists was proved by
R. L. Graham and B. L. Rothschild. The actual answer is believed to be 6, but the best bound proved is
of one color will be forced. That an answer exists was proved by
R. L. Graham and B. L. Rothschild. The actual answer is believed to be 6, but the best bound proved is
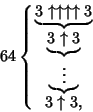
See also Arrow Notation, Chained Arrow Notation, Skewes Number
References
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 61-62, 1996.
Gardner, M. ``Mathematical Games.'' Sci. Amer. 237, 18-28, Nov. 1977.