|
|
|
An equation derived by Kronecker: ![]()
|
|
|
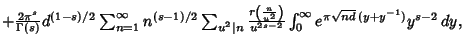
|
where
References
Borwein, J. M. and Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity.
New York: Wiley, pp. 296-297, 1987.
Selberg, A. and Chowla, S. ``On Epstein's Zeta-Function.'' J. Reine. Angew. Math. 227, 86-110, 1967.