|
|
|
The Minimal Surface having a Helix as its boundary. It is the only Ruled Minimal Surface other than the Plane (Catalan 1842, do Carmo 1986). For many years, the helicoid remained the only known example of a complete embedded Minimal Surface of finite topology with infinite Curvature. However, in 1992 a second example, known as Hoffman's Minimal Surface and consisting of a helicoid with a Hole, was discovered (Sci. News 1992).
The equation of a helicoid in Cylindrical Coordinates is
| (1) |
| (2) |
| (3) | |||
| (4) | |||
| (5) |
| (6) | |||
| (7) | |||
| (8) |
| (9) |
| (10) | |||
| (11) | |||
| (12) |
| (13) |
| (14) |
| (15) |
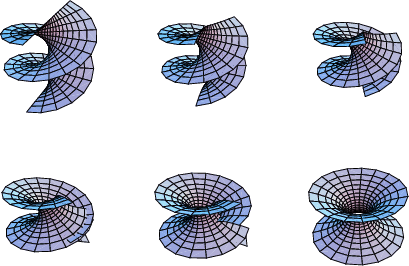
The helicoid can be continuously deformed into a Catenoid by the transformation
| (16) | |||
| (17) | |||
| (18) |
If a twisted curve ![]() (i.e., one with Torsion
(i.e., one with Torsion ![]() ) rotates about a
fixed axis
) rotates about a
fixed axis ![]() and, at the same time, is displaced parallel to
and, at the same time, is displaced parallel to ![]() such that the speed of displacement is always
proportional to the angular velocity of rotation, then
such that the speed of displacement is always
proportional to the angular velocity of rotation, then ![]() generates a Generalized Helicoid.
generates a Generalized Helicoid.
See also Calculus of Variations, Catenoid, Elliptic Helicoid, Generalized Helicoid, Helix, Hoffman's Minimal Surface, Minimal Surface
References
Catalan E. ``Sur les surfaces réglées dont l'aire est un minimum.'' J. Math. Pure Appl. 7, 203-211, 1842.
do Carmo, M. P. ``The Helicoid.'' §3.5B in
Mathematical Models from the Collections of Universities and Museums (Ed. G. Fischer).
Braunschweig, Germany: Vieweg, pp. 44-45, 1986.
Fischer, G. (Ed.). Plate 91 in
Mathematische Modelle/Mathematical Models, Bildband/Photograph Volume.
Braunschweig, Germany: Vieweg, p. 87, 1986.
Geometry Center. ``The Helicoid.''
http://www.geom.umn.edu/zoo/diffgeom/surfspace/helicoid/.
Gray, A. Modern Differential Geometry of Curves and Surfaces.
Boca Raton, FL: CRC Press, p. 264, 1993.
Kreyszig, E. Differential Geometry. New York: Dover, p. 88, 1991.
Meusnier, J. B. ``Mémoire sur la courbure des surfaces.'' Mém. des savans étrangers 10 (lu 1776), 477-510, 1785.
Peterson, I. ``Three Bites in a Doughnut.'' Sci. News 127, 168, Mar. 16, 1985.
``Putting a Handle on a Minimal Helicoid.'' Sci. News 142, 276, Oct. 24, 1992.
Wolfram, S. The Mathematica Book, 3rd ed. Champaign, IL: Wolfram Media, p. 164, 1996.
|
|
|
© 1996-9 Eric W. Weisstein