|
|
|
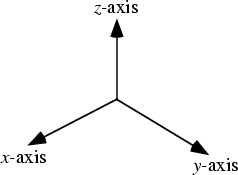
Cartesian coordinates are rectilinear 2-D or 3-D coordinates (and therefore a special case of Curvilinear Coordinates)
which are also called Rectangular Coordinates. The three axes of 3-D Cartesian coordinates, conventionally denoted the
x-Axis-, y-Axis-, and z-Axis (a Notation due to
Descartes ![]() ) are chosen to be linear and mutually Perpendicular. In 3-D, the coordinates
) are chosen to be linear and mutually Perpendicular. In 3-D, the coordinates ![]() ,
, ![]() , and
, and ![]() may lie anywhere in the Interval
may lie anywhere in the Interval
![]() .
.
The Scale Factors of Cartesian coordinates are all unity, ![]() . The Line Element is given by
. The Line Element is given by
| (1) |
| (2) |
| (3) |
| (4) |
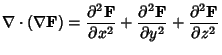 |
|||
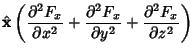 |
|||
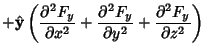 |
|||
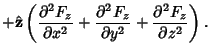 |
(5) |
| (6) |
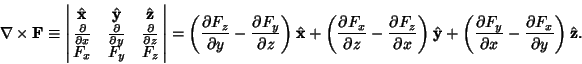 |
(7) |
![$\displaystyle \left[\begin{array}{c} {\partial\over\partial x}\left({{\partial ...
...ial u_y\over\partial y}+{\partial u_z\over\partial z}}\right)\end{array}\right]$](c1_326.gif) |
|||
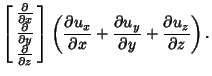 |
(8) |
Laplace's Equation is separable in Cartesian coordinates.
See also Coordinates, Helmholtz Differential Equation--Cartesian Coordinates
References
Arfken, G. ``Special Coordinate Systems--Rectangular Cartesian Coordinates.'' §2.3 in
Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 94-95, 1985.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, p. 656, 1953.
|
|
|
© 1996-9 Eric W. Weisstein