|
|
|
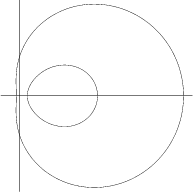
A curve consisting of two ovals which was first studied by Descartes ![]() in 1637. It is the locus of a point
in 1637. It is the locus of a point ![]() whose distances from two Foci
whose distances from two Foci ![]() and
and ![]() in two-center Bipolar Coordinates satisfy
in two-center Bipolar Coordinates satisfy
| (1) |
| (2) |
| (3) |
|
|
|
|
|
(4) |
| (5) | |||
| (6) |
| (7) |
| (8) |
| (9) |
References
Cundy, H. and Rollett, A. Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., p. 35, 1989.
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 155-157, 1972.
Lockwood, E. H. A Book of Curves. Cambridge, England: Cambridge University Press, p. 188, 1967.
MacTutor History of Mathematics Archive. ``Cartesian Oval.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Cartesian.html.
|
|
|
© 1996-9 Eric W. Weisstein