|
|
|
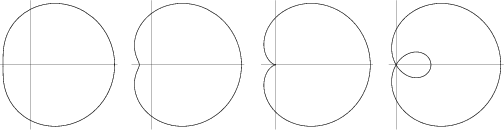
The limaçon is a polar curve of the form
If ![]() , we have a convex limaçon. If
, we have a convex limaçon. If ![]() , we have a dimpled limaçon. If
, we have a dimpled limaçon. If ![]() , the limaçon
degenerates to a Cardioid. If
, the limaçon
degenerates to a Cardioid. If ![]() , we have limaçon with an inner loop. If
, we have limaçon with an inner loop. If ![]() , it is a
Trisectrix (but not the Maclaurin Trisectrix) with inner loop of Area
, it is a
Trisectrix (but not the Maclaurin Trisectrix) with inner loop of Area
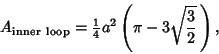
See also Cardioid
References
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 113-117, 1972.
Lee, X. ``Limacon of Pascal.''
http://www.best.com/~xah/SpecialPlaneCurves_dir/LimaconOfPascal_dir/limaconOfPascal.html
Lee, X. ``Limacon Graphics Gallery.''
http://www.best.com/~xah/SpecialPlaneCurves_dir/LimaconGGallery_dir/limaconGGallery.html
Lockwood, E. H. ``The Limaçon.'' Ch. 5 in A Book of Curves. Cambridge, England: Cambridge University Press,
pp. 44-51, 1967.
MacTutor History of Mathematics Archive. ``Limacon of Pascal.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Limacon.html.
Yates, R. C. ``Limacon of Pascal.'' A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 148-151, 1952.