The Scale Factors are
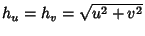 ,
, 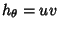 and the separation functions are
and the separation functions are
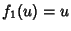 ,
,  ,
, 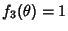 , given a Stäckel Determinant of
, given a Stäckel Determinant of
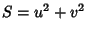 . The Laplacian is
. The Laplacian is
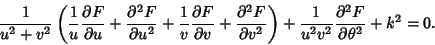 |
(1) |
Attempt Separation of Variables by writing
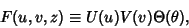 |
(2) |
then the Helmholtz Differential Equation becomes
![\begin{displaymath}
{1\over u^2+v^2}\left[{V\Theta \left({{1\over u}{dU\over du}...
...{dV\over dv}+{d^2V\over dv^2}}\right)}\right]+k^2 UV\Theta =0.
\end{displaymath}](h_1054.gif) |
(3) |
Now divide by 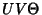 ,
,
![\begin{displaymath}
{u^2v^2\over u^2+v^2}\left[{{1\over U}\left({{1\over u}{dU\o...
...ght)}\right]+{1\over \Theta} {d^2\Theta\over d\theta^2}+k^2=0.
\end{displaymath}](h_1056.gif) |
(4) |
Separating the 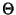 part,
part,
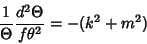 |
(5) |
![\begin{displaymath}
{u^2v^2\over u^2+v^2}\left[{{1\over U}\left({{1\over u}{dU\o...
...{{1\over v}{dV\over dv}+{d^2V\over dv^2}}\right)}\right]= k^2,
\end{displaymath}](h_1058.gif) |
(6) |
so
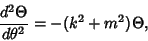 |
(7) |
which has solution
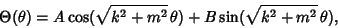 |
(8) |
and
![\begin{displaymath}
\left[{{1\over U}\left({{1\over u}{dU\over du}+{d^2 U\over d...
...}+{d^2 V\over dv^2}}\right)}\right]-k^2{u^2+v^2\over u^2v^2}=0
\end{displaymath}](h_1061.gif) |
(9) |
![\begin{displaymath}
\left[{{1\over U}\left({{1\over u}{dU\over du}+{d^2 U\over d...
...V\over dv}+{d^2 V\over dv^2}}\right)-{k^2\over v^2}}\right]=0.
\end{displaymath}](h_1062.gif) |
(10) |
This can be separated
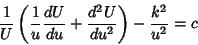 |
(11) |
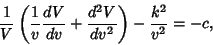 |
(12) |
so
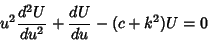 |
(13) |
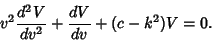 |
(14) |
References
Arfken, G. ``Parabolic Coordinates
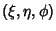 .'' §2.12 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 109-111, 1970.
.'' §2.12 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 109-111, 1970.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York:
McGraw-Hill, pp. 514-515 and 660, 1953.
© 1996-9 Eric W. Weisstein
1999-05-25
![]() ,
, ![]() and the separation functions are
and the separation functions are
![]() ,
, ![]() ,
, ![]() , given a Stäckel Determinant of
, given a Stäckel Determinant of
![]() . The Laplacian is
. The Laplacian is
![]() .'' §2.12 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 109-111, 1970.
.'' §2.12 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 109-111, 1970.