|
|
|
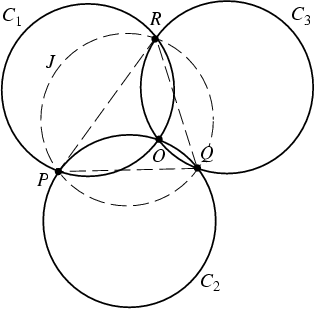
Let three equal Circles with centers ![]() ,
, ![]() , and
, and ![]() intersect in a single point
intersect in a single point ![]() and intersect
pairwise in the points
and intersect
pairwise in the points ![]() ,
, ![]() , and
, and ![]() . Then the Circumcircle
. Then the Circumcircle ![]() of
of ![]() (the so-called Johnson
Circle) is congruent to the original three.
(the so-called Johnson
Circle) is congruent to the original three.
See also Circumcircle, Johnson Circle
References
Emch, A. ``Remarks on the Foregoing Circle Theorem.'' Amer. Math. Monthly 23, 162-164, 1916.
Honsberger, R. Mathematical Gems II.
Washington, DC: Math. Assoc. Amer., pp. 18-21, 1976.
Johnson, R. ``A Circle Theorem.'' Amer. Math. Monthly 23, 161-162, 1916.