A 1-variable Knot Polynomial denoted 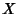 or
or 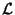 .
.
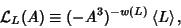 |
(1) |
where
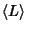 is the Bracket Polynomial and
is the Bracket Polynomial and 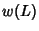 is the Writhe of
is the Writhe of 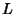 . This Polynomial is invariant
under Ambient Isotopy, and relates Mirror Images by
. This Polynomial is invariant
under Ambient Isotopy, and relates Mirror Images by
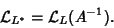 |
(2) |
It is identical to the Jones Polynomial with the change of variable
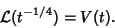 |
(3) |
The 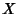 Polynomial of the Mirror Image
Polynomial of the Mirror Image 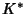 is the same as for
is the same as for 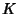 but with
but with 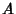 replaced by
replaced by 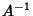 .
.
References
Kauffman, L. H. Knots and Physics. Singapore: World Scientific, p. 33, 1991.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() or
or ![]() .
.