|
|
|
Gamma Functions of argument ![]() can be expressed in terms of Gamma Functions of smaller arguments. From the definition of the Beta Function,
can be expressed in terms of Gamma Functions of smaller arguments. From the definition of the Beta Function,
| (1) |
| (2) |
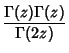 |
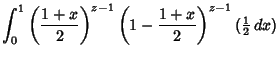 |
||
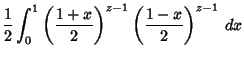 |
|||
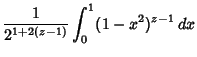 |
|||
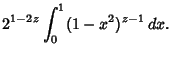 |
(3) |
| (4) |
| (5) |
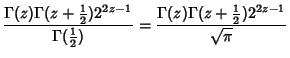 |
|||
| (6) |
See also Gamma Function, Gauss Multiplication Formula
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 256, 1972.
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 561-562, 1985.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 424-425, 1953.