|
|
|
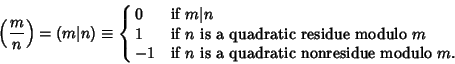
See also Jacobi Symbol, Kronecker Symbol, Quadratic Reciprocity Theorem
References
Guy, R. K. ``Quadratic Residues. Schur's Conjecture.'' §F5 in
Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 244-245, 1994.
Shanks, D. Solved and Unsolved Problems in Number Theory, 4th ed. New York: Chelsea, pp. 33-34 and 40-42, 1993.